The Marginal Rate of Technical Substitution

In the long run, all inputs (and therefore, costs) are variable. This provides the producer an opportunity to react and substitute away from a factor of production that becomes relatively more expensive and mitigate the increase in costs.

Given the following production function:

X = f(L, K)

we can write (via total differentiation):

ΔX = MP_LΔL + MP_KΔK,

that is, changes in output (in the long run) are measured as the sum of changes in labor input (via the marginal productivity of labor) and / or changes in capital (via the marginal productivity of capital). Holding output constant (ΔX = 0, as we would on a given production isoquant), we can derive:

0 = MP_LΔL + MP_KΔK,

or

ΔK / ΔL = MP_L / MP_K,

This last result defines the slope of a Production Isoquant 'ΔK / ΔL' as being equal to the ratio of marginal productivities 'MP_L / MP_K'. This ratio is also known as the Marginal Rate of Technical Substitution (MRTS) or the rate by which one factor may be substituted for another.

Using the Cobb-Douglas production function as a particular mathematical function we can derive:

X = AL^αK^β

and

MP_L = αAL^α-1K^β = αX/L

MP_K = βAL^αK^β-1 = βX/K

and the Marginal Rate of Technical Substitution:

MRTS = MP_L / MP_K = αK / βL = (α / β)(K / L.

In the case of Cobb-Douglas technologies, the MRTS is proportional to the ratio of factor inputs used. For example if &alpah; was equal to 0.75 and β equal to 0.25, then the MRTS is equal to: 3(K/L).

Profit Maximization

Given a profit equation:

π = P[f(L, K)] - (wL + rK)

where the term in the square brackets represent output via the production function [X = f(L,K)]. The first-order conditions are:

dπ/dL = P[MP_L] - w = 0

and

dπ/dK = P[MP_K] - r = 0.

If we solve for 'P' (the market price of the output) in both equations and set them equal to each other we have:

MP_L / w = MP_K / r

or

MP_L / MP_K = w/r

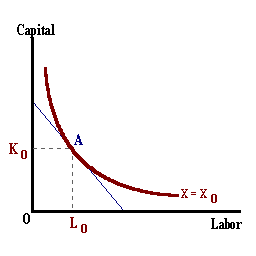
The condition for profit maximization (or cost minimization) is where the MRTS is just equal to the ratio of factor-input prices ('w' & ' r'). This condition is known as a Producer Optimum in the Long Run and defined for a given level of output 'X₀' as shown at point ' A' in figure 1 below.

Figure 1, A Long Run Producer Optimum

Note that in the case of the Cobb-Douglas production function, the Producer Optimum may be defined as:

αK / βL = (w/r)

or the cost-minimizing combination of these two inputs is:

K / L = (β/α) (w/r)

or

K = (β/α) (w/r) L.

For example if the specific Cobb-Douglas production function is estimated as:

X = 1000L^0.80K^0.20

and the wage rate 'w' is equal to $20.00 and cost per unit of capital 'r' is equal to $10.00,

K = (1/4) (2/1)L

or

K = (1/2)L

The firm would use capital and labor in a 1:2 ratio (2 units of labor for each unit of capital). This makes sense since labor is four-times as productive as a unit of capital (α=0.80 and β =0.20) but only twice as expensive.

Long Run Costs

A cost equation in the long run (all costs are variable) may be written as:

C = wL + rK

or

solving for K = f(L) -- slope-intercept form:

K = C_o/r + (w/r)L.

This expression is known as the Iso-Cost line or line of equal costs with a slope defined by the ratio of factor prices (w/r) and shown in the above diagram (figure 1) as the navy-blue line.

Changes to output levels would require more of both inputs such that costs would increase. As long as the ratio of factor prices does not change, the ratio of factor-intput use will also not change.

An increase in one of the factor prices will lead the profit-maximizing (cost-minimizing) firm to substitute away from the factor that has become more expensive and towards the relatively cheaper factor-input. For example, an increase in the wage rate will lead the firm to find a different combination of inputs in order to produce the same level of output. In this case the firm will substitute away from labor (L₀ --> L₁) and towards capital (K₀ --> K₁) as shown in the diagram below:

Figure 2, An Increase in the Wage Rate

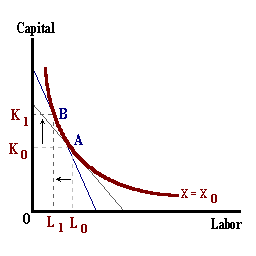
In the case of a Cobb-Douglas technology this substitution is possible such that costs at point 'B' have increased relative to point 'A' but by a smaller amount than if substitution were not possible.

ΔCosts = (Δ_[+]w) [L₁ - L₀] + (r)[K₁ - K₀]

but if:

(Δ_[+]w) [L₁ - L₀] + (r)[K₁ - K₀] < (Δ_[+]w)[L₀] + (r)[K₀]

This would occur with a Leontief technology where factor-inputs must always be used in fixed proportion. In this case the costs of production would increase in direct proportion to the increase in the wage rate:

ΔCosts = (Δw)L₀

This helps explain why factor price increases are strongly resisted in industries governed by a Leontief technology.