• To act as a medium of exchange to facilitate the payment of income and purchase of goods and services.
• To act as a unit of account--a measure by which all prices are established, and
• To act as a store of value -- that is to alter the timing of spending decisions relative to earning income.

Because of the dual role of money as a medium of exchange and store of value; there are several economic variables affect the desire to hold this type of financial asset.

Money can be narrowly defined as anything that may be used for purchasing goods and services or more broadly to include anything of value that may be used for trade. Two common definitions as established by monetary authorities are M₁ and M₂:

• M₁ = Currency + Demand Deposits (checking accounts or current accounts)
• M₂ = M₁ + Time Deposits (simple interest-bearing savings accounts)

The first measure is known as the narrow definition of money which represents components that are readily accepted as payments for goods or to satisfy debts. The second measure is known as a broader definition which includes savings accounts that can easily be converted into currency or demand deposits.

Individuals typically hold cash balances (money) to allow for making transactions (that is buying goods and services and the paying of bills and other obligations. The volume of these transactions tends to be proportional to that individual's level of income thus the demand for these cash balances in support of transactions needs will also be proportional to income 'Y'.

M_d = k(Y)

This is best understood by looking at the cash balances held by an individual over time. Assume that a person is paid a monthly salary of $3000 and paid twice a month. On the 1^st and 15^th of each month, this person is paid $1500 which is held as cash or as a deposit in a checking (current) account. Over the days that follow these cash balances are run-down as this person buys goods and services or pays his monthly bills such that towards the end of a pay period, his cash balances are close to $0. However, at the beginning of the next pay period $1500 is received and his cash balances are restored. Thus at the beginning of a pay period this person is holding (demanding) $1500 and towards the end of the pay period he is holding some amount close to $0. On average this person has cash balances of about $750 [($1500-0)/2].

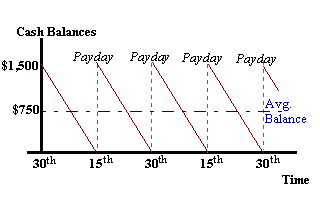
This represents part of his individual demand for cash balances or money. By aggregating over all individuals and institutions in the economy we can derive the aggregate demand for money as the sum of individual demands. With an increase in income (either for an individual or in the aggregate) we would expect that more is held such that the average amount held over time increases.

M_d^T = f_[+](Y) -- Note: 'Y' = Nominal GDP in the aggregate

One might question the notion that at the end of a pay period, cash balances are equal to $0. Cash balances not used for transactions represent a source for savings (a surplus of funds). The individual might choose to keep these "savings" in the form of currency of on deposit in a checking account. But by making this choice, the individual is giving up the opportunity to earn some form of return (or yield) on these funds in the form of interest, profits, or rents. As yields rise, the opportunity cost of holding cash balances also increases inducing the individual to minimize his cash holdings. The individual can do this by buying an alternative financial asset in the form of a time deposit (or certificate of deposit), share of stock, or a bond. When one of these assets is purchased (or demand deposit balances are converted to time deposit balances) the individual's cash balances are reduced. We can amend our expression for money demand as follows:

M_d = f (Y_[+], i_[-])

We can therefore state that money demand is directly proportional to Nominal GDP, and inversely related to market interest rates and yields on different financial assets. Thus economic performance in the real sector (changes in income) or activity in financial markets (buying and selling of stocks, bonds, and related financial instruments) can affect the demand for money/cash balances.

This cost relationship can be expressed as follows: 

min C = b(Y/M) + M(i) 

• b = the cost of making a single cash transaction 

(i.e., withdrawals from a checking or savings account or conversion into stocks and bonds) 
• Y = Income. 
• i = a market-determined  interest rate or yield.
• M = the size of Cash Balances. 

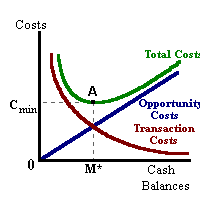
Minimizing the sum of these costs implies finding the lowest point on the total cost curve in the above diagram. This point occurs at point 'A' corresponding with optimal balances of 'M*' This optimal value may also be found by using calculus and taking the derivative of the cost function  with respect to M and setting the result equal to zero: 

dC/dM = -bY/M² + i = 0 

bY/M² = i

M* = (bY/i)^1/2 = (bY)^1/2(i)^-1/2

In more general form, a money demand equation with similar functional form could be written as:

M_d* = A(Y)^ρ (i)^β